Integration
We have to integrate functions of the form
. The rule here is to add one to the power and divide by the new power, never forgetting that we must add a constant.
This extends to any combination of sums and multiples of powers of
For example, remembering that
which may be simplified further.
This adding of one to the power and dividing by the new power generalises to fractional and negative powers. For example,
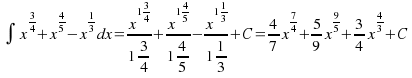
If we have limits, values of 
between which the integral takes place, then the answer is just a number.
If the integral has limits then we have no need for C, since we have C from the first bracket take C from the second bracket.
There is one case where the above rule does not work.
We cannot divide by zero but we can still evaluate the integral. This is a special case, discussed in C2.