Any 'normal' continuous function![]() can be expressed as a polynomial. A 'normal' function has a smooth curve with no corners, so that we can differentiate it as many times as we need to with no restrictions. To say that the function is continuous on an interval means that the graph of the function may be drawn without lifting pen from the paper. The graph below is continuous but not differential. It cannot be represented by a Maclaurin series because it is not differentiable on any interval containing 0.
can be expressed as a polynomial. A 'normal' function has a smooth curve with no corners, so that we can differentiate it as many times as we need to with no restrictions. To say that the function is continuous on an interval means that the graph of the function may be drawn without lifting pen from the paper. The graph below is continuous but not differential. It cannot be represented by a Maclaurin series because it is not differentiable on any interval containing 0.
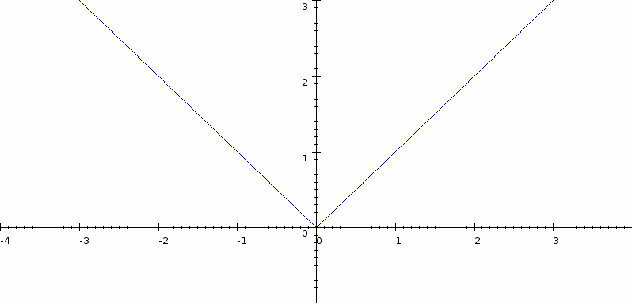
The polynomial that represents f(x) may be infinite – that is, it may have an infinite number of terms - and if the function is not one to one, so that each value on the range corresponds to only one value in the domain, then the domain may have to be restricted to an interval on which the function is one to one. Given this we may express![]() as a sum of powers of
as a sum of powers of![]() multiplied by differentials of
multiplied by differentials of![]()
![]()
In this expression![]() means
means![]() has been differentiated
has been differentiated![]() times then
times then![]() substituted.
substituted.
The are several points to notes.
Putting![]() eliminates all but the first term on the right hand side and the left hand side is
eliminates all but the first term on the right hand side and the left hand side is![]()
Differentiating both sides and substituting![]() gives
gives![]() on the left hand side. The first term on the right hand side disappears, the second term becomes
on the left hand side. The first term on the right hand side disappears, the second term becomes![]() and all succeeding terms disappear because
and all succeeding terms disappear because![]() so both sides equal
so both sides equal![]() if both sides are differentiated. In general if we differentiate both sides
if both sides are differentiated. In general if we differentiate both sides![]() times then put
times then put![]() then both sides equal
then both sides equal![]()
If![]() is a polynomial of degree
is a polynomial of degree![]() then all but the first
then all but the first![]() terms vanish. If
terms vanish. If![]() then:
then:
![]()