The momentum of a body of mass m moving with velocity v is given by mv. Notice that velocity is used and not speed, so we must take the direction of the body into account. It is useful to form the habit of taking right as positive and left as negative directions respectively – this is in fact the convention.
Momentum is conservation any interaction of any number of bodies. The interaction may be at a distance so that the bodies do not touch, or the bodies may come into contact with each other – there is a collision. For two bodies with masses![]() and
and![]() and velocities
and velocities![]() and
and![]() in collision,
in collision,![]() or equivalently,
or equivalently,
momentum before collision = momentum after collision.
If we have one of the velocities of one of the bodies after the collision we can use this equation to find the other.
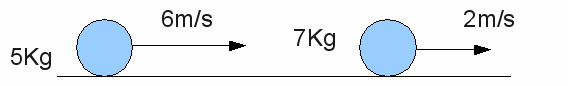
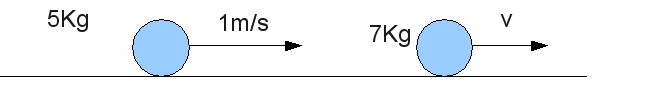
Example:The diagrams below illustrate the collision of two particles of mass 5Kg and 7Kg. In the first diagram both particles are moving to the right, so both velocities are positive.
Before
After
The initial momentum, from the first diagram, is![]()
The final momentum, from the second diagram, is![]()
Because momentum is conserved, these two are equal:
![]() to 1 dp.
to 1 dp.
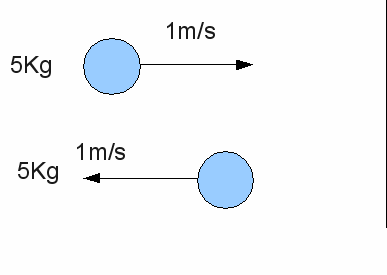
The diagram below shows a ball of mass 5Kg hitting a wall and rebounding with equal speed but opposite velocity. It would appear that momentum is not conserved, but the diagram below is not a complete picture. The Earth has been excluded. If we included the Earth in our analysis we would find momentum is conserved.