There are two well known andfamiliar equations for the resistance in a circuit:
![]() (1)for resistances in series
(1)for resistances in series
Proof:
![]()
The current in each resistoris the same - ![]() -and the voltage across each resistor adds to the total voltage acrossthe two resistors -
-and the voltage across each resistor adds to the total voltage acrossthe two resistors - ![]() Usethe equation
Usethe equation ![]() foreach resistance individually and the whole circuit to get,
foreach resistance individually and the whole circuit to get,
![]() anddivide by the common factor
anddivide by the common factor ![]() toget
toget ![]()
and ![]() (2)for resistances in parallel.
(2)for resistances in parallel.
Proof:
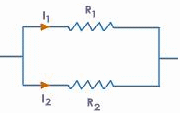
The voltage across eachresistor is the same - ![]() -and the current through each resistor adds to the total currentthrough the two resistors -
-and the current through each resistor adds to the total currentthrough the two resistors - ![]() Usethe equation
Usethe equation ![]() foreach resistance individually and the whole circuit to get,
foreach resistance individually and the whole circuit to get,
![]() anddivide by the common factor
anddivide by the common factor ![]() toget
toget ![]()
More fundamental however isthe equation ![]() (3)which introduces the resistivity
(3)which introduces the resistivity
![]() ofa material. The resistivity is a fundamental property of thematerial, ranging from
ofa material. The resistivity is a fundamental property of thematerial, ranging from ![]() fora conductor like silver to
fora conductor like silver to ![]() fora resistor like wood.
fora resistor like wood.
The resistivity equation (3)is in fact a consequence of the other two, since having tworesistances in series is equivalent two having twice the length ofwire, so we may say:
Resistance is directlyproportional to length.
The equation for twoidentical resistances in parallel implies that two resistances inparallel halves the total resistance. This is equivalent to doublingthe cross sectional area. This implies that the resistance isinversely proportional to the area. We may write down the equation:
![]() whereK is a constant of proportionality which we call the resistivity andlabel
whereK is a constant of proportionality which we call the resistivity andlabel ![]()