Three graphical concepts are very useful – the intercepts (particularly the y – intercept), the gradient of the graph at a point and the area under the graph.
The intercept is a point on an axis where the graph crosses.
If the graph is a line and the y – intercept is zero then the quantity represented on the y axis is said to be directly proportional to the quantity represented on the x – axis.
The gradient of a straight line graph is the increase in the y – value as we move along the graph divided by the increase in the x – value. For a straight line graph the gradient is constant. For example, the gradient below is![]() Notice the triangle drawn is as large as possible. The graph illustrates Hooke's Law.
Notice the triangle drawn is as large as possible. The graph illustrates Hooke's Law.
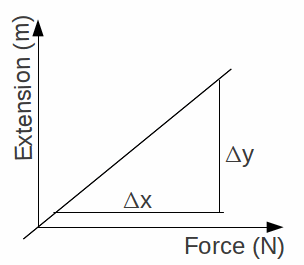
Often the area under the graph represents a useful quantity. The area under the graph above represents the energy needed to stretch the spring. The area above is a triangle so is equal to![]() Often the area can only be estimated, by counting squares for example.
Often the area can only be estimated, by counting squares for example.