The sum of a sequence is said to converge if![]()
There are two very useful theorems for deciding whether or not the sum of a sequence converges.
The Ratio Test
If there exists![]() such that
such that![]() for
for![]() then
then![]() converges. The test says nothing about sequences such that
converges. The test says nothing about sequences such that![]() where
where![]() means
means ![]() from below.
from below.
Proof:
Suppose first that![]() for all
for all![]()
![]()
Hence![]()
Example:![]()
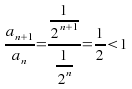 therefore
therefore![]() converges.
converges.
The Comparison Test
We can prove convergence of divergence for some sequences by comparing the sequence with a 'standard' sequence, the sum of which which either converges or diverges.
For example suppose![]() and
and![]() then
then![]()
The proof is obvious.
Standard convergent sequences include
![]()
Standard divergent sequences include
![]()
Example:![]()
![]() which is a standard convergent sequence therefore
which is a standard convergent sequence therefore![]() converges.
converges.