If the set of real numbers![]() is countable then there exists a one to one correspondence between the set of natural numbers
is countable then there exists a one to one correspondence between the set of natural numbers![]() and
and![]() Conversely if
Conversely if![]() is not countable then no such one to one correspondence exists. We can prove that no such correspondence exists by showing that for each set of real numbers
is not countable then no such one to one correspondence exists. We can prove that no such correspondence exists by showing that for each set of real numbers![]() a real number
a real number![]() exists which does not belong to the sequence.
exists which does not belong to the sequence.
Define a sequence of closed intervals![]() such that
such that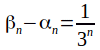 and
and![]() for
for![]()
Divide the closed interval![]() into intervals of equal length
into intervals of equal length![]() and
and![]() and choose one not containing
and choose one not containing![]() Call that interval
Call that interval![]()
Divide![]() into three equal sets and choose one not containing
into three equal sets and choose one not containing![]() Call that interval
Call that interval![]()
Keep going in the same manner until we have the closed interval ![]() of length
of length![]() which does not contain the point
which does not contain the point![]() Let
Let![]() denote the common intersection of all the intervals
denote the common intersection of all the intervals![]()
![]() for each
for each![]() but
but![]() because
because![]() We have a sequence of real numbers
We have a sequence of real numbers![]() not containing
not containing![]() so that
so that![]() is not countable.
is not countable.