It is often useful to remove the units from an equation. We can see which physical mechanisms are more important and the equation to be solved is simpler. We scale all variables so that the variables become dimensionless. We can solve the equation relative to properties of the system e.g. the period.
To make an equation dimensionless one must do the following:
-
Identify all the independent and dependent variables, e.g.
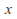 having dimension
having dimension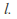
-
Replace each of them with a quantity scaled relative to a characteristic unit e.g.
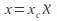 where
where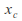 is constant and has dimension
is constant and has dimension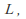 and
and is variable and dimensionless;.
is variable and dimensionless;. -
Rewrite the system of equations in terms of their new dimensionless quantities.
-
Divide through by the coefficient of the highest order derivative term;.
-
Choose the scale factors so that as many terms as possible have coefficient 1.
Example: Make![]() dimensionless.
dimensionless.
1. The independent variable is![]() and the dependent variable is
and the dependent variable is![]()
2. Let![]() and
and![]() where
where![]() and
and![]() are constants to be defined later.
are constants to be defined later.
3. Since![]() we have
we have
![]()
4. The coefficient of the highest order term is![]() and dividing by this leads to
and dividing by this leads to![]()
5. Choose![]() and
and![]()
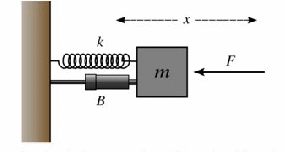
Example: Make![]() dimensionless where
dimensionless where![]() is the equilibrium position.
is the equilibrium position.
This is the equation of the system below.
1. The independent variable is![]() and the dependent variable is
and the dependent variable is![]()
-
Make
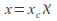 and
and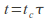 then follow the procedure in 3 above to get
then follow the procedure in 3 above to get -
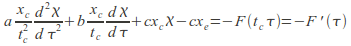
Divide through by the leading coefficient![]() to give
to give![]()
![]()
5. Choose![]() and add 1 to both sides to give
and add 1 to both sides to give![]() where
where![]()