Centres of Mass, Summation Method

For a uniform regular body with symmetry eg a cube, flat triangle, sphere, the centre of mass is in the middle. Unfortunately, most bodies are not regular or uniform. To find the centre of mass of an arbitrary body we must use one of the formulae,

oror same variation of one of these formulae.

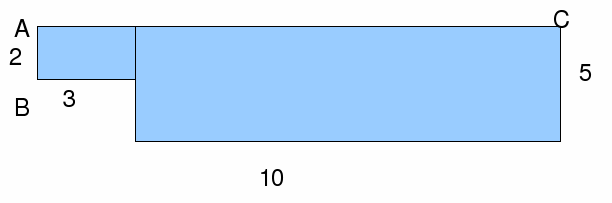
For example, we find the centre of mass of the object below. For illustrative purposes, take the density of the second one as half the density of the first.

Take moments about AB:

Take moments about AC:

The centre of gravity isright from AB andbelow AC.

The process for the Integral Method is similar.