Pascal's triangle provides a quick and easy way to calculate the coefficients of the binomial expansion,
The entries in the table are calculated by adding the entties in each table immediately above to the left and right, with the exceptions of the first and last entires in each row, which are both 1 as show for the entry 10 indicated below.
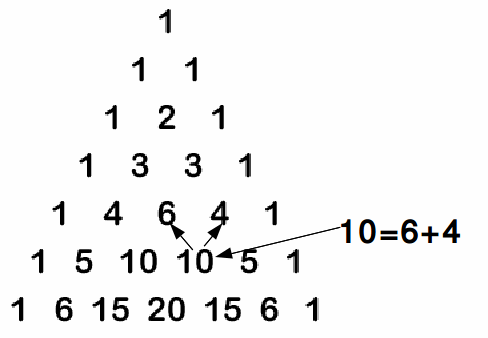
Sequences of numbers in Pascal;s triangle have interesting properties.
Entries in the nth row add to give
so that from the fourth row,
The table is symmetrical, reading the same from the left as from the right, a consequence of the fact that
Entries from the first diagonal, shown below, from the sequence of integers,
Entries from the second diagonal form the triangle numbers
Entries from the second diagonal,
Similar formulae exist for other diagonals.
