\[x\]
and \[y\]
axes. We can find the distanceof the points of intersection of points on the \[x\]
axis and points on the \[y\]
axis by first finding the points of intersection, then using the distance formula for the distance between two points.Suppose we have the circle with equation given by
\[(x-2)^2+(y-4)^2=25\]
. This circle has centre \[(2,4)\]
and radius 5.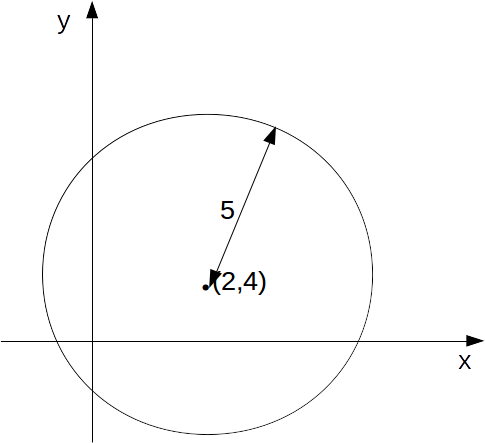
\[x\]
axis when \[y=0\]
.\[(x-2)^2+(0-4)^2=25 \rightarrow x-2 =\pm \sqrt{25-16}= \pm 3 \rightarrow x=2-3=-1,2+3=5\]
.The points of intersection with the
\[x\]
axis are \[(-1,0),(5,0)\]
.The circle intersects the
\[y\]
axis when \[x=0\]
.\[(x-2)^2+(y-4)^2=25 \rightarrow y-4 =\pm \sqrt{25-4}= \pm \sqrt{21} \rightarrow y=4-\sqrt{21}, \: 4+\sqrt{21}\]
.The points of intersection with the
\[y\]
axis are \[(0, 4-\sqrt{21}),(0,4+\sqrt{21})\]
.The distance between the points closest to the origin,
\[(-1,0)\]
and \[(4-\sqrt{21},0)\]
is \[d=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}=\sqrt{(-1-0)^2+(0-(4-\sqrt{21})^2}=1.157\]
to three decimal places. 