The remainder theorem states that when a polynomial![]() is divided by a linear expression
is divided by a linear expression![]() the remainder is
the remainder is![]()
Example: When![]() is devider by
is devider by![]() substitute
substitute![]() to obtain the remainder
to obtain the remainder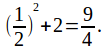
We can prove it quite easily by performing long division of![]() by
by![]() to obtain the quotient
to obtain the quotient![]() and remainder
and remainder![]() We can write
We can write
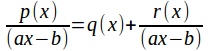
or equivalently
![]() (1)
(1)
If the degree of![]() is
is![]() then the degree of
then the degree of![]() is
is ![]() and the degree of
and the degree of![]() must be one less than the degree of
must be one less than the degree of![]() ie the degree of
ie the degree of![]() is 0 so
is 0 so![]() is a constant. We can write
is a constant. We can write
![]()
Now substitute![]()
![]()
so that![]() as required.
as required.
