A line, which is a two dimensional object, is fixed by two points on it – two dimensions, two points. The equation of a line can be written given by
– this is the cartesian form of the line. The cartesian form of a plane is
where
and
are constants To find the equation of a plane we need three points. Each point determines an equation in
We solve these simultaneous equations to find the constants
in terms of
and write down the equation of the plane. Finally we cancel the constant d which appears throughout.
Example: A plane passes through the three points
and
Find the equation of the plane.
Substituting the first point
into the equation of the planegives
Similarly the second and third give
and
We solve the simultaneous equations,
(1)
(2)
(3)
(1)+(3) gives
Sub
into (2) to give
Sub
andinto (1) to give
The equation of the plane is then
Cancel the factorto give
and clear all the fractions to give the final answer
There is an alternative form for the equation of a plane to terms of vectors:
where
and
are parameters and
is a point in the plane. For the plane give above we can find
and
by subtracting points in the plane from each other:
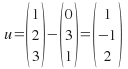and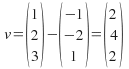 
.
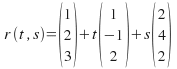The vector form is not unique since any points in the plane can be used.