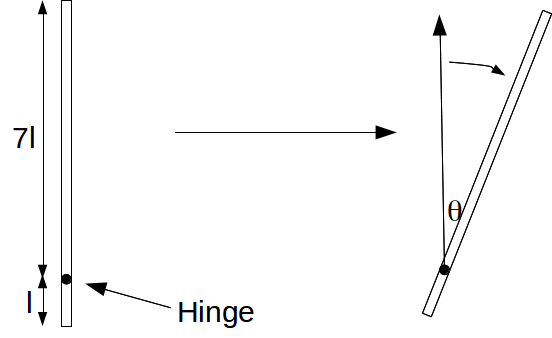
If a rod AB of length![]() and mass
and mass![]() is smoothly hinged at a point a distance
is smoothly hinged at a point a distance![]() from the end A and, held with the end B above A and then given a small push so that it is free to rotate, we can apply conservation of energy to obtain the angular velocity
from the end A and, held with the end B above A and then given a small push so that it is free to rotate, we can apply conservation of energy to obtain the angular velocity![]() of the rod after it has turned through an angle
of the rod after it has turned through an angle![]()
The moment of inertia of a rod of length![]() and mass M about its centre is
and mass M about its centre is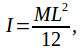 so the moment of inertia of the rod above about its centre is
so the moment of inertia of the rod above about its centre is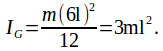
The parallel axis theorem states that if it is pivoted about a parallel axis a distance![]() (equal to
(equal to![]() in this case) from the first, the moment of inertia about that point is
in this case) from the first, the moment of inertia about that point is![]()
The kinetic energy of the rad at an time is![]()
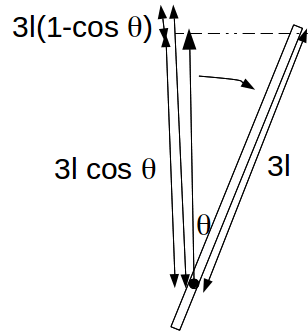
When the rod has turned through an angle![]() the centre of mass has fallen a distance
the centre of mass has fallen a distance![]() (see the diagram which shows the changing position of the centre of mass).
(see the diagram which shows the changing position of the centre of mass).
The increase in kinetic energy is equal to the loss in gravitational potential energy.
![]() (1)
(1)
Taking moments about the hinge give![]()
where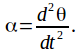 This is the same equation as obtained by differentiating (1) with respect to t.
This is the same equation as obtained by differentiating (1) with respect to t.
![]()
![]()
Divide by![]() and put
and put![]() The result is
The result is![]()
