Solving Second Order Constant Coefficient Homogeneous

Second order differential equations arise naturally as a result of applying Newton's second law of motion. If no external forces act then the equation may take the form

whereis a drag or resistance term andis a restoring force.

If there is no resistance term then the equation becomes- the equation of simple harmonic motion - and this equation has the solution

If there is no restoring force the the equation becomes

We can write this equations as

Integration givesWe can solve this equation by separation of variables

Integration givesand solving for x gives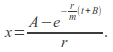

If there are both resistance and restoring terms then there are three possibilities.

We can substituteso thatandThe equation becomes

We can divide by(since an exponential is never zero) to obtain

This is a quadratic equation with roots

Ifthen there are two distinct real rootsandand

Ifthen there is one rootand

In both of these cases,may increase without limit ifis positive, and the motion is non – oscillatory.

Ifthen there are two distinct complex roots

and and

This last solution forrepresents oscillatory motion. Ifthe oscillations decay and if the oscillations increase in size.