The Continuity and Bernoulli Equations
Fluid dynamics describes the bulk properties of fluid flow - pressure, speed, head etc. The most important equations of the continuity equation:![]() illustrated below.
illustrated below.
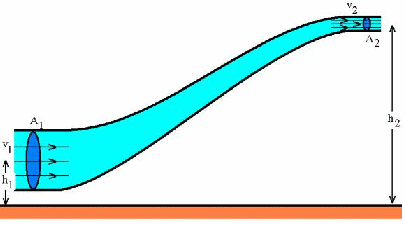
The other important fluid dynamics equation is Bernoulli's equation, derived from the Principle of Conservation of Energy. This is,
![]()
Where P=Pressure (Pa or![]() )
)
![]() = density of fluid
= density of fluid
![]() =acceleration due to gravity
=acceleration due to gravity
![]() = speed of fluid
= speed of fluid
![]() = height of fluid above a reference point.
= height of fluid above a reference point.
It is Bernoulli's equation that explain how planes fly.
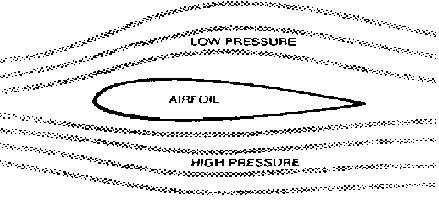
As air passes over the aerofoil, molecules passing over the top must travel faster than molecules travelling over the bottom, since the distance over the top is greater. Therefore, according to Bernoulli's equation, if we assume constant height and density, which we usually can, the pressure underneath the wing must be greater than pressure over the wing. There is thus a net force on the wing pushing up.
During a hurricane the difference between the low pressure inside and the high pressure outside can produce enough force to lift the roof off a house. This is another illustration of Bernoulli's principle.