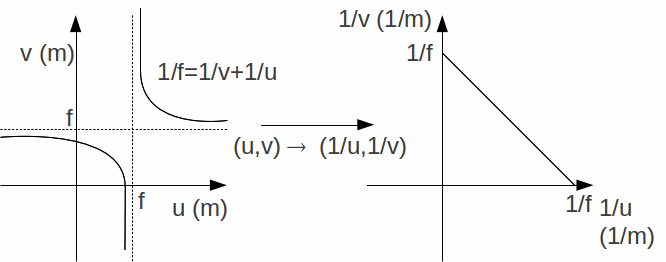Not every equation naturally gives rise to a straight line. If we have variables![]() and
and![]() related by
related by![]() or
or![]() and
and![]() related by
related by![]() then graphs of
then graphs of![]() against
against![]() or
or![]() against
against![]() will not result in a straight line. We are however not restricted to plot
will not result in a straight line. We are however not restricted to plot![]() against
against![]() or
or![]() against
against![]() We can often rearrange an equation into a form which will give a straight line if we plot a suitable function of one variable against a suitable function of the other. In order to do this we need to:
We can often rearrange an equation into a form which will give a straight line if we plot a suitable function of one variable against a suitable function of the other. In order to do this we need to:
-
Identify which symbols in the equation are variables and which are constants.
-
The symbols that correspond to
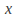 and
and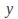 must be variables and the symbols that correspond to
must be variables and the symbols that correspond to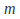 and
and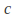 must be constants.
must be constants. -
If a variable is cubed, square rooted or the reciprocal, log or exponential is taken, the result is still a variable and may still be used to label one of the axes.
-
Any function of the readings may be used to label the axes, since the result is still a variable.
-
Sometimes the physical quantities use the same symbols as in our notation e.g.
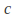 is used to denote the speed of light. Do not get these confused.
is used to denote the speed of light. Do not get these confused.
For the equation![]() above, taking natural logs results in a straight line.
above, taking natural logs results in a straight line.
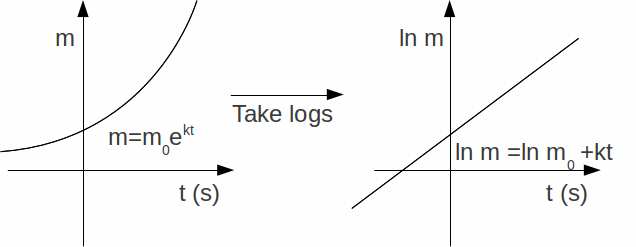
For the equation![]() above, plotting
above, plotting![]() against
against![]() results in a straight line with gradient -1 and
results in a straight line with gradient -1 and![]() intercept
intercept![]() since
since![]()