Understanding the process of beta decay requires the introduction of a virtually undetectable particle – the neutrino. This particle is needed because there appears to be missing energy – the energy of the beta particle emitted is not quite equal to the difference in energy between the nucleus before and after the decay. We can find the missing energy using
for the nucleus before the decay and the decay products – the nucleus after the decay and the beta particle.
For example tritium decays into helium with the emission of a beta particle.
The products,
and
together weigh less than the tritium nucleus, the difference being
This implies using conservation of energy that the beta particle should have 19.5 keV of kinetic energy. In fact this is found to be an upper limit for the kinetic energy, with most emitted beta particles having less energy than this, and the average kinetic energy for this particular decay only being about 10 keV. All beta decays follow this pattern, with an upper limit for the kinetic energy of the emitted beta particle and missing energy. A typical distribution of beta particle energy is shown below.
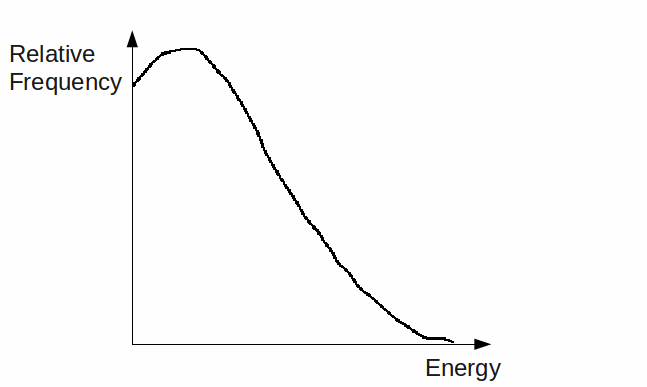
The neutrino is very hard to detect so must have certain properties.
It must be electrically neutral.
Because the lower limit for the energy carried away by a neutrino is close to zero (and may be zero), the mass of a neutrino must be zero (or close to zero).
The full equation for the decay given above is
Positron decays are also possible. The positron is the antiparticle of the beta particle. During positron decay, a proton decays into a neutron with the emission of a neutrino (as opposed to an antineutrino for beta decay). For example
