A set of objects is enumerable or countable if it can be counted, or more precisely, if it can be put into a one to one correspondence with the set of natural numbers
Some sets which are 'obviously' not enumerable turn out to be, and also surprisingly, sets whih are larger or smaller than setn , containing setn as a proper subset, or a proper subset of setn , turn out to be capable of being put into a one to one correspondence with elements of setn , so are in a very real sense, the same size as setn .
The set of even numbers E can be put into a one to one correspondence with elements of setn .
If
then there exists
and there is one such element of
for each and every element of
and vice versa so the mapping
with 
is a one to one mapping fromto
Much more surprisingly, the set of rational numbers (fractions) is enumerable. There are many more rational numbers that natural numbers since every natural number can be written as a fraction, but not vice versa. To demonstrate enumerability, construct the table shown below.
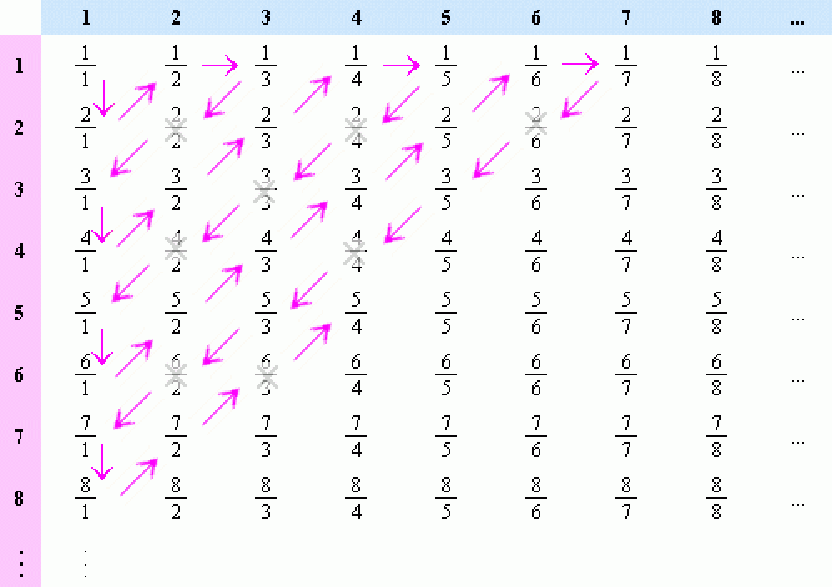
With natural numbers in the margins, start in the upper left hand corner and work diagonally. The first rational number is
the second is
the third is
the fourth is
and so on. Every rational number appears somewhere is the table (the rational number
appears at position
in the table) so the rational numbers are enumerable.