Proof That Normal Distribution has a Point of Inflexion One Standard Deviation From the Mean

If the random variableis described by a normal distributionthen the distribution ofis descibed by a probability density function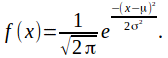

Differentiating this gives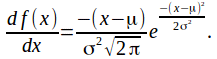

Differentiating again (using the product rule) gives

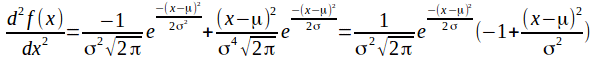
Since 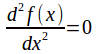if and only if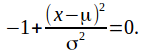

Henceor

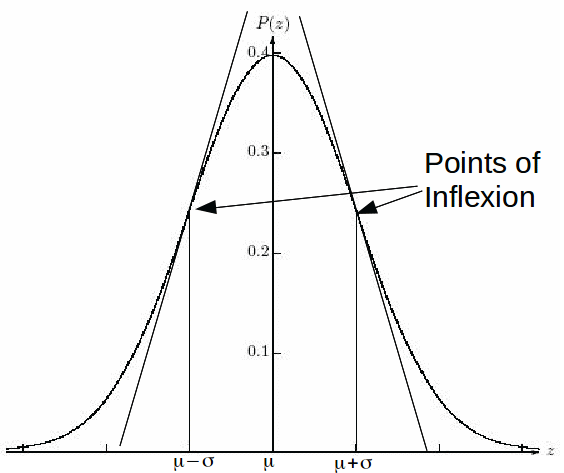
The points of inflexion are shown below (note that a point of inflexion is such that the curve crosses a tangent at a point).