Classificatin of Open Channel Flow

Consider the steady, uniform flow of an inviscid, incompressible liquid in an open channel with a rectangular cross section of constant width. If the width isand the depth isthe volume flow ratewhereis the speed of the liquid.is constant from the continuity equation, since the liquid does not accumulate in any part of the channel. Ifis the volume flow rate per unit width then

We apply Bernoulli's equation along a streamline in the free surface of the liquid in a flat bottomed channel, givingwhereis the atmospheric pressure, assumed constant. Since the density is also constant we can subtractfrom each side, givingDividing bygiveswhereis called the specific energy.

Ifis constant thensoor

The last equation is a cubic in h, so may have 3 distinct real roots or one. If there are three roots for this particular equation, one of the roots is negative, so physically meaningless, so for givenandthere are either one or two possible values ofThere are three possible cases.

1. If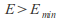two flows are possible at different depths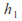and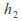corresponding to speeds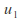and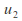are possible.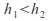and since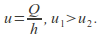The first is described as shallow and fast or supercritical, the second as deep and slow or subcritical.

2. If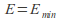the flow is unique. The liquid flows with depth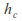and spee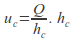dand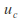are the critical speed and critical depth respectively.

3. If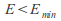then no flow is possible.

All the cases are illustrated below.

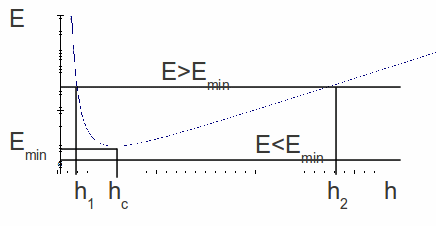
Since the critical depthoccurs at a minimum of the specific energy function, we can differentiateand equate to zero to find

The minimum value of the specific energy is then