Minkowski diagrams allow the path of particles through spacetime to be visualized. Though spacetime is four dimensional,
Minkowski diagrams display only two of these, one of which is the time axis, which points upwards. The path of a particle is a line or a curve in the direction of the positive time axis. The path of a light ray has special significance. The equation of a light ray is
This line has gradient
Any other line must have gradient of magnitude greater than 1, reflecting the fact that the speed of a particle can be at most the speed of light, and must be less than 1 for a matter particle.
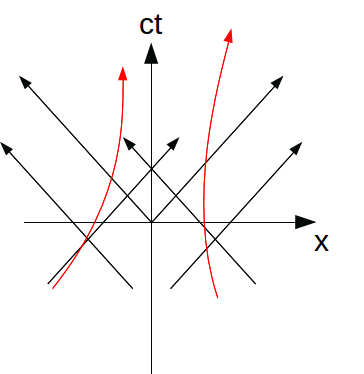
The lines shown in black are possible paths of light rays and the curves in red are possible paths of matter particles. Any path passing from below the x axis to above the x axis goes from past (t<0) to future (t>0). A path which passes through the origin can be considered to pass through the the origin of an intertial frame at
Different inertial frames moving with constant velocity relative to each other may be represented on the same Minkowski diagram. If an inertial frame O' with axes
is moving with velocity
along the x axis of the inertial frame O above then the Lorentz transformation gives the coordinates in O' of any events with coordinates
in O. They are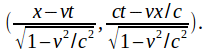
On the x' axis,
and on the
axis
so the equations of the x' axis in O is
and the equation of theaxis in O is
