A function![]() is odd is
is odd is![]() and even if
and even if![]()
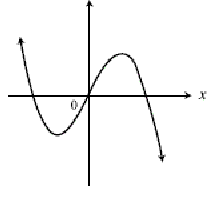
The function above is odd. Every odd function has rotational symmetry order two about the origin, so we can rotate it by![]()
![]() is odd since
is odd since![]()
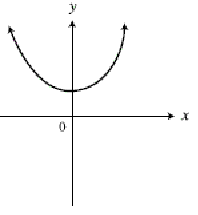
The function above is even. Every even function is symmetric with respect to the y – axis, so it will be the same graph after reflection in the y - axis.
![]() is even since
is even since![]()
Every arbitrary function can be expressed as a sum of odd and even functions.
![]() is an odd function since
is an odd function since![]()
![]() is an even function since
is an even function since![]()
Products of odd and even functions obey similar laws to the sign laws for multiplying positive and negative numbers.
even*even=even
odd*odd=even
odd*even=odd
even*odd=odd
There are also laws for composing odd and even functions
even(even)=even since![]()
odd(odd)=odd since![]()
even(odd)=even since![]()
odd(even)=even since![]()
