Boxplots

Boxplots are used to visually represent a data set consisting of a list of numbers. It displays the lower and upper quartiles, the median, and any outliers or extreme values. Suppose we have an ordered list of numbers representing the lengths, in cm, of some worms:

2, 3, 7, 7, 12, 14, 16, 17, 17, 17, 34

There list is eleven numbers long.

The median is thethe sixth number. 14.

The lower quartile,is found by dividing the length of the list by 4 then rounding up to give the place of the number in the list.which rounds up to 3. The third number is 7.

The upper quartile,is found by dividing the length of the list by 4 and multiplying by 3 then rounding up to give the place of the number in the list.which rounds up to 9. The ninth number is 17.

Now we have to find the limits below and above which outliers lie.

The interquartile range,is the difference between the lower and upper quartiles. For the list above the IQR=17-7=10.

The lower limit isAny length less than the lower limit is an outlier.

The upper limit isAny length more than the upper limit is an outlier.

The lower limit isNegative lengths are obviously impossible, so there are no outliers at the lower end.

The upper limit isThe longest snail is longer than the upper limit so the length of 34 cm is an outlier.

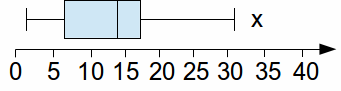
Notice that the verical bars at the end of each line are either to the extreme values which are not outliers, or, if there are outliers, to the limits beyond which outliers lie.