A probability distribution is usually defined in terms of it's probability distribution function (if continuous), the probability that it takes a value in a certain range, or probability mass function (if discrete), the probability that it takes a certain value. Sometimes it is more convenient to define it in terms of a cumulative distribution function.
If the probability density function for
where
may be finite or
and
may be finite or
is given by
then the cumulative distribution function, cdf, such that
is given by
if
is continuous
if X is discrete.
Example: The continuous quantityis uniformly distributed over the interval
The probability distribution is
The cumulative distribution function is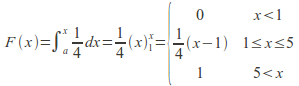
Example: The probability distribution of a random variableis given in the following table. Construct the cumulative distribution function.
|
|
0 |
1 |
2 |
3 |
4 |
5 |
|
|
0.10 |
0.05 |
0.00 |
0.25 |
0.15 |
0.45 |
To find the cumulative distribution function, add up the entries in therow as you go along, to give
|
|
0 |
1 |
2 |
3 |
4 |
5 |
|
|
0.10 |
0.15 |
0.15 |
0.40 |
0.55 |
1.00 |
Conversely given a cumulative distribution function we can find the probability distribution function by differentiation, or by subtraction each
from the previous one to give
in the case of a discrete distribution.
Example: If
then
Example:is given in the following table.
|
|
0 |
1 |
2 |
3 |
4 |
5 |
|
|
0.20 |
0.25 |
0.35 |
0.40 |
0.75 |
1.00 |
is given in the table below.
|
|
0 |
1 |
2 |
3 |
4 |
5 |
|
|
0.20 |
0.25-0.20=0.05 |
0.35-0.25=0.10 |
0.40-0.35=0.05 |
0.75-0.40=0.35 |
1.00-0.75=0.25 |
