Student's t - Distribution

Ifobservationsare taken from a normal distribution with meanandvariancethe sample meanfollowsa normal distribution

In practice however thepopulation varianceisrarely known and must also be estimated. If the varianceisestimated as(isunbiased forsothat)from a large sample of sizethen,the sample mean is approximately normally distributed,theaccuracy of the approximation improving with increasinguntil,at thet distribution is identical to the normal distribution.

When n is small however, thenormal approximation given above is not accurate enough and we mustuse Student's t – distribution:

If a random sampleisselected from a normal distribution with meanandunknown variance thehasadistribution,where

In fact there is not asingle t distribution. There is a whole family, each with anassociated number of degrees of freedom,hencethe labelusedabove.

Thedistributionis an approximation to the normal distribution for each value of

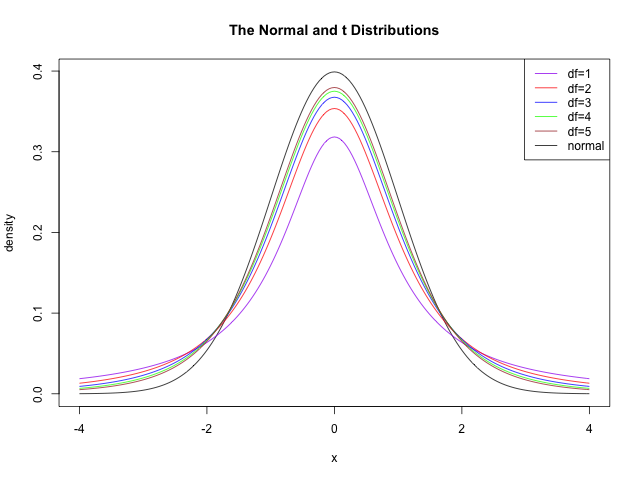
Like the normaldistribution, the t – distribution is symmetrical and unimodal (theprobability density function has one peak, as in the diagram above),and also like the normal distribution, calculations invoving the tdistribution from first principles is not elementary and must be donefrom tables.