Apparatus:
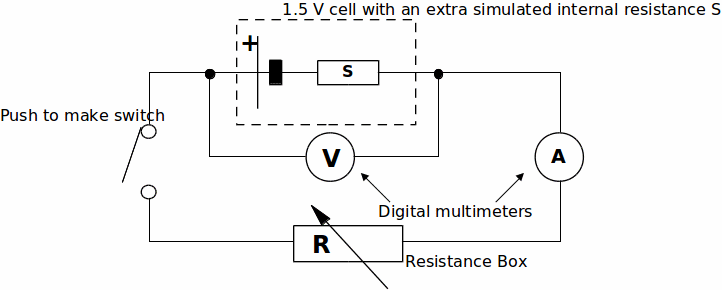
1.5V cell, Mounted and taped unknown resistor labelled 'S', push to make switch, 0 to 1000 OHM resistance box, two digital multimeters, wires.
Diagram:
The internal resistance,
of a
cell is typically about
To prevent damage to the cell, you are going to pretend that the resistor labelled 'S' is also inside the cell.
The dashed lines in the above diagram will represent the boundary of the 'modified' cell.
The resistor box will act as the external resistance,
of this circuit.
Procedure:
1. Adjust the resistor box so that it has a resistance of
2. Set up the circuit as above.
3. Measure the current through the cell (I) and the potential difference across the cell (V)
for values of
and
IN THIS ORDER
DO NOT PRESS DOWN THE SWITCH LONGER THAN NECESSARY
4. Tabulate the values of
&
in a suitable table.
5. Draw a graph of
/ volts (
- axis) against
/ A (
- axis). It should be a straight line of negative gradient.
(NOTE: Plot current in amperes!)
6. Measure theintercept. This should equal the EMF of the cell.
7. Measure the gradient of your graph. This will equal the negative of the combined resistance of resistor 'S' and the actual internal resistance r of the cell.
8.equals the power being transferred by the cell to the external resistance
Draw a graph of/ watts (y - axis) against/
(- axis). It should be a curve with a maximum.
Approximately what value ofyields the maximum power transfer?
How does this compare with the 'modified' internal resistance?
THEORY:
For a complete circuit
where
The voltmeter reading,is equal to the external potential difference,
Therefore
and so
rearranged is
The equation is now in the form
is equivalent to
to
to
the gradient and
to
the y-axis intercept.
Hence the gradient of theagainstgraph is equal to
and the intercept with the y-axis to