Heat Capacities of Ideal Gases at Constant Volume

For an ideal gas the internal energy depends only on the temperaturewhere

is the number of mols andis the Universal Gas Constant,

When the temperature increases by a small amountthe corresponding change in internal energy is

The specific heat capacity of a substance is defined asso for a gas withmols we see the heat capacity at constant volume isand the molar heat capacity at constant volume, labelledis

In fact the above equation only holds for gases whose particles are single atoms. It is more accurate to say that the molar hear capacity for an ideal gas isper degree of freedom. This allows us to generalise to gases made up of molecules of two or more atoms.

For a monotomic gas there are 3 degrees of freedom: up and down, forwards and backwards, left and right.

For a diatomic gas there are two extra, 'vibrational' degrees of freedom and two extra 'rotational' degrees of freedom, each contributing an extra to the molar heat capacity at constant volume. For a diatomic gas,

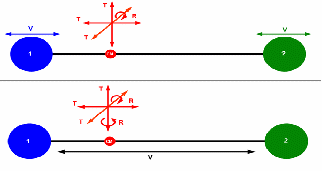
It must be noted though that the vibrational degrees of freedom only manifest above certain temperatures. For lower temperatures