Area Between Intersecting Squares

The diagram shows squares of 6cm and 10cm. A corner of the 10cm square is at the centre of the 6cm square.

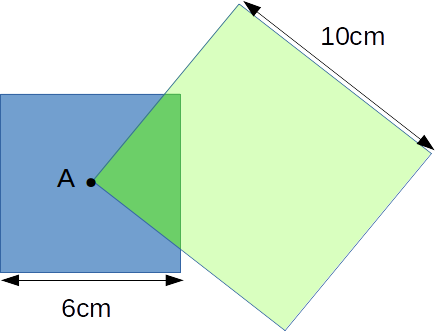
What is the area of the intersection of the two squares, shaded in turquoise? We can rotate the large green square four times around the point A, so that it entirely covers the blue square.

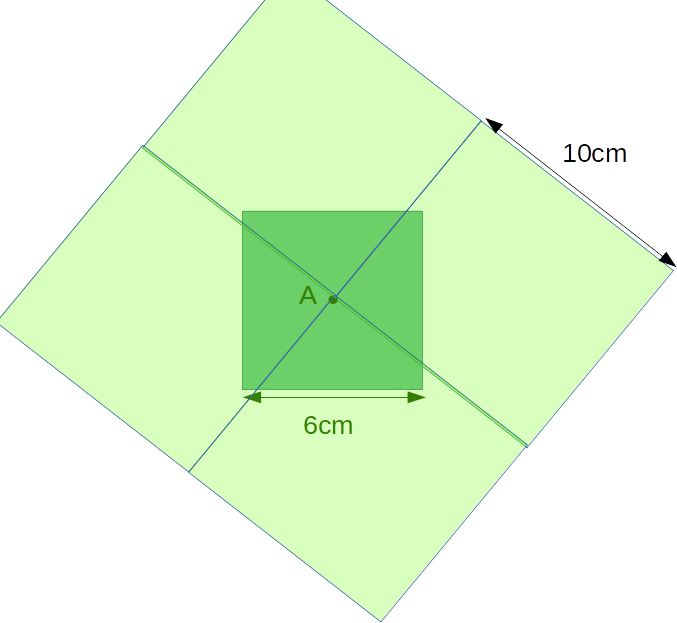
There are now four turquoise shapes, each with the same are. so the area of each must be a quarter of the area of the small blue square.
The turquoise area is  .