You may be familiar with the picture of Archimedes in his bath just before he jumped out and ran down the street shouting, “Eureka!” - “I've found it!” He was presented with the problem of testing the purity of a royal crown – was it pure gold or not?
He solved the problem by finding the density of the metal of which the crown was made. When he was climbing into his bath the water overflowed. He realized that he could find the volume of the crown by measuring how much water it displaced when immersed. The weight could be measured with a pair of scales then the density found using the equation
However, this is not Archimedes Principle:
When a body is immersed or floating in a fluid, the upthrust on the body is equal to the weight of fluid displaced.
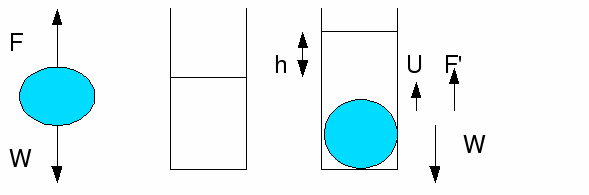
When the ball is dropped into the liquid the volume of liquid displaced is equal to the volume of the ball. The effective weight is reduced by the amount of the upthrust ie F becomes F'=W-U. But the upthrust is equal to the weight of fluid displaced, %rho gV where %rho = density of liquid, g=9.81 and V=the volume of fluid displaced=Volume of the ball. We may write 
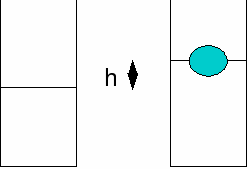
The upthrust is still equal to the weight of fluid displaced and we may still use the equation 
but V is not now the volume of the ball. We must calculate V using the equation V=h*cross sectional area of liquid .