\[\sum_i \mathbf{F}_i =0\]
.From Newton's First Law, we know that an object constantly at rest and an object moving with constant velocity in a straight line are in equilibrium.
Since forces are vector quantities, zero resultant force mean no net force acting in any direction. For problems in 2 dimensions it is sufficient to show that the forces balance in any two non parallel directions.
Translational equilibrium does not mean that the same as 'being at rest', neither does an object being at an equilibrium point mean that the object is in equilibrium.
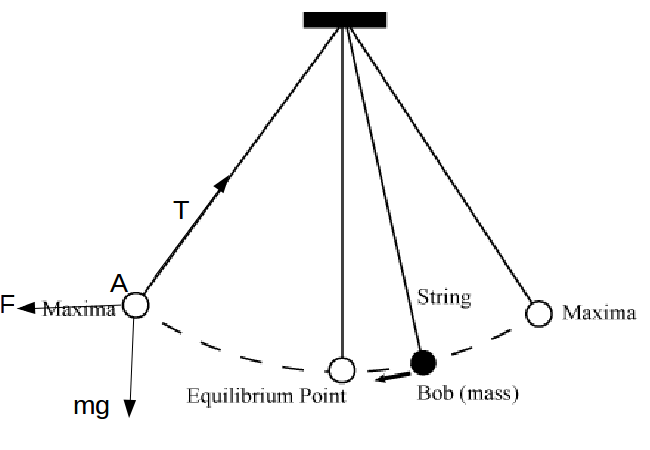At the point A the three forces \[\mathbf{F}, \: \mathbf{T}, \: m \mathbf{g}\]
are in equilibrium. When \[\mathbf{F}\]
is removed, the pendulum is momentarily at rest but the forces are unbalanced at not in equilibrium. At the equilibrium point, the forces are momentarily balanced so the pendulum is momentarily in translational equilibrium - but only for a moment.