Electrons can be described by waves, with each electron in a particular state having a particular wavelength and energy. It should be possible therefore to express electrons in atoms, with their discrete energy levels, in terms of waves. Schrodingers model pictures the electron moving around the atom, with a whole number of wavelengths fitted into one orbit, the electron moving in three dimensions. More technically, the electrons are described by standing waves whose wavefunctions that fit the boundary conditions in the atom. Some possible standing waves of electrons are shown schematically below. The continuous curves represent the wave representing the electron, and the dotted lines represent the wave describing the atom half a cycle later.
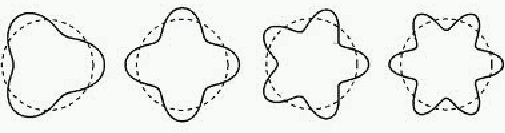
The standing waves on a string have definite wavelengths but this is not true for the electron in the atom. The radius of the electron orbit is not fixed. If the radius of the orbit increases, so must the wavelength to fit the same number of waves into the orbit. This also means that the speed of the electron decreases with increasing radius, and the kinetic energy also decreases. This means that the wavefunctions that fit the boundary conditions (of having a whole number of wavelengths in the orbit for example) have particular shapes. The wavefunctions are in three dimensions and can be quite complicated. Some are shown below.

The images are made up of dots, with each dot represneting an electron found at that point. The probability of finding the electron increases with the number of dots in the above diagrams. These pictures are experimental but we can explain them using the wavefunction. The probability of finding an electron in a small volume dV of space is the product of this small volume with the wavefunction 2.
