\[-1\]
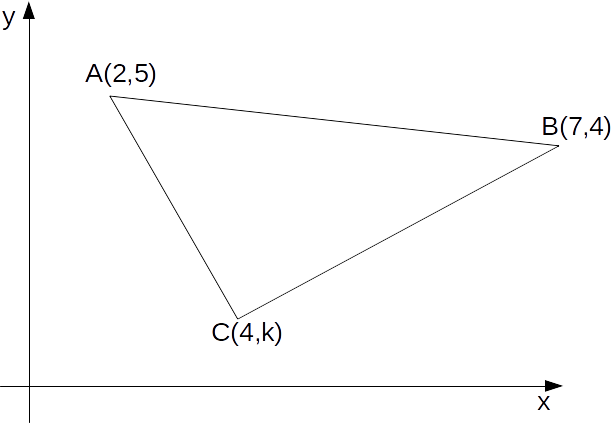
, then the triangle is right angled, We can also use this condition to find the coordinates of a vertex. The triangle below has a right angled at \[C(4,k)\]
and we want to find the value of \[k\]
.
\[-1\]
The gradient of AC is
\[\frac{k-5}{4-2}=\frac{k-5}{2}\]
The gradient of CB is
\[\frac{4-k}{7-4}=\frac{4-k}{3}\]
Hence
\[\frac{k-5}{2} \frac{4-k}{3} =-1\]
Rearranging gives
\[(k-5)(4-k) =-6 \rightarrow -k^2+9k-20=-6 \rightarrow k^2-9k+14=0 \]
This factorises to give
\[(k-7)(k-2)=0\]
.Hence
\[k=7, \: 2\]
.Obviously, from the diagram above
\[k=2\]
and the point C has coordinates \[(4,2)\]
