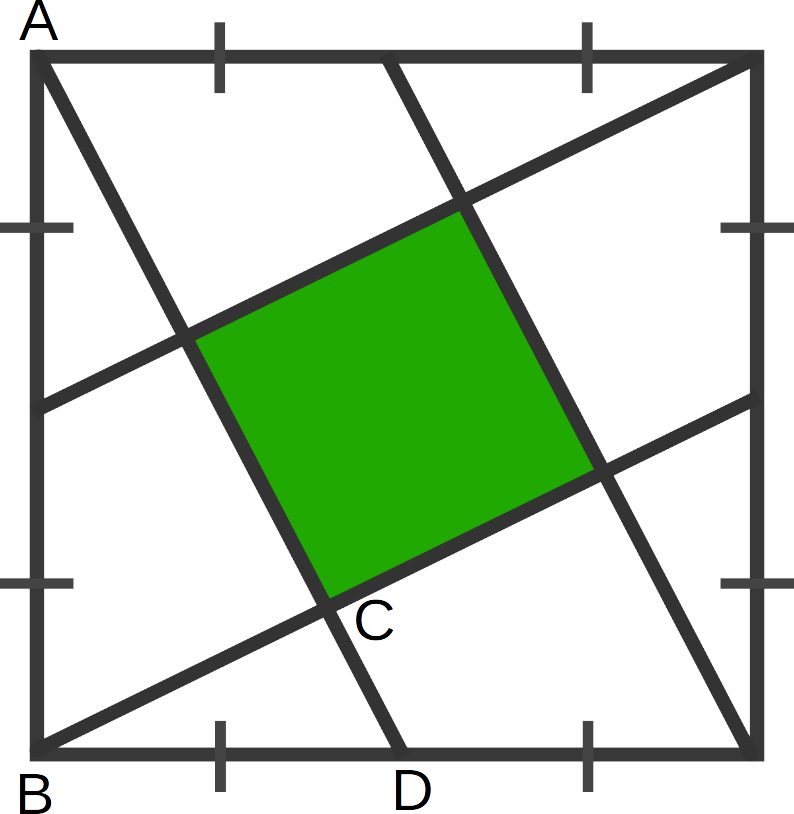
\[\angle BAC= \alpha \rightarrow \angle ABC=90- \alpha \rightarrow \angle CBD= 90-(90- \alpha)= \alpha \rightarrow \angle BCD=90- \alpha\]
.If
\[Ab=x\]
then \[BC=\frac{x}{2}\]
and the area of triangle ABD is \[\frac{1}{2} \times x \times \frac{x}{2}=\frac{x^2}{4}\]
The areas of triangles ABC and BDC are in the ratio
\[(2)^2= 4\]
so Area ABC is \[\frac{4}{1+4} \times \frac{x^2}{4}=\frac{x^2}{5}\]
.Then the area of the green square is
\[x^2 - 4 \times \frac{x^2}{5}=\frac{x^2}{5}\]
.The area of the Green square is one fifth the area of the big square.
