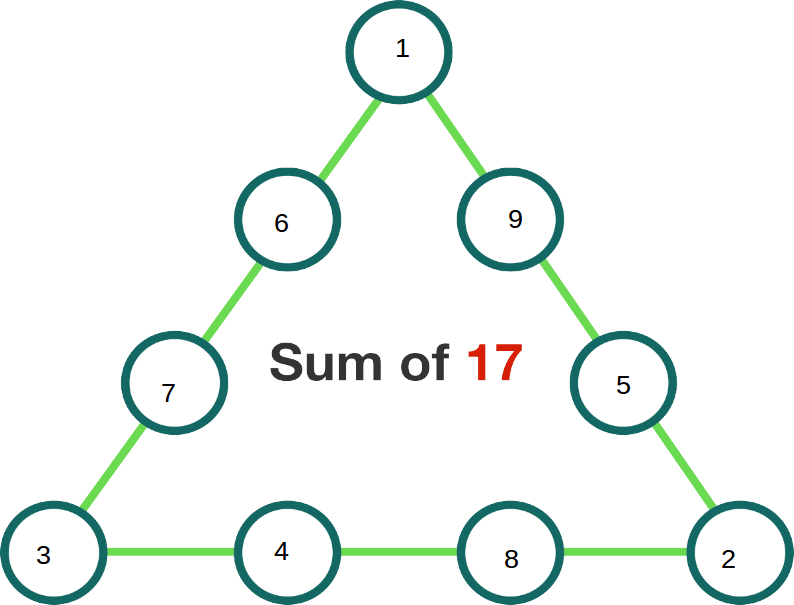
Arrangle the numbers 1 to 9 around a triangle so that the numbers on each side - including the corners - add up to 17.
There is always some symmetry about these problems. The numbers 1 to 9 add up to 15, but the sides of the triangle (with corners counted twice) is 51. The difference is 6, so the corners add up to 6, and this can only be so if the corners are 1, 2 and 3. The number 7, 8 and 9 must go along seaparate sides. After some trial and error, the answer is: