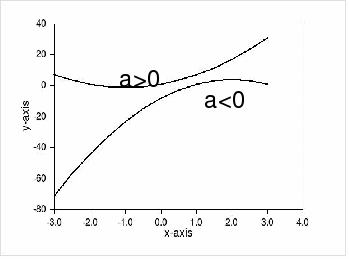
To find the minimum or maximum of a quadratic we complete the square expressing the function in the form![]()
If![]() the minimum will be where
the minimum will be where![]() so
so![]() and the minimum is at
and the minimum is at![]()
If![]() the maximum will be where
the maximum will be where![]() so
so![]() and the maximum is at
and the maximum is at![]()
For example, to find the minimum of
![]() complete the square to get
complete the square to get![]() then the minimum is at
then the minimum is at![]()
To find the maximum of![]() complete the square to get
complete the square to get![]() then the maximum is at
then the maximum is at![]()
We might also have to find the maxima of reciprocal quadratics such as![]()
The quadratic here can have no roots if it is to have a maximum, or else at those roots we would have![]() which has no value, and close to those roots the graph would tend to
which has no value, and close to those roots the graph would tend to![]() As before we complete the square to get
As before we complete the square to get![]() To maximise y we have to minimise the denominator ie minimise
To maximise y we have to minimise the denominator ie minimise![]() This has a minimum at
This has a minimum at![]() hence
hence![]() has a maximum at
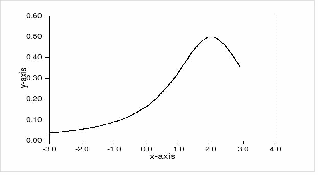
has a maximum at![]() This is illustrated below. If the numerator were negative we would follow the same procedure, completing the square but now find a minimum, in this case at
This is illustrated below. If the numerator were negative we would follow the same procedure, completing the square but now find a minimum, in this case at![]()