Summary of Terms Used in Probability

Two events A and B are mutually exclusive if they cannot both occur, either at the same time, or one after the other, depending on the circumstances. If they are mutually exclusive andandare the probabilities of events A, B or both A and B occurring respectively, then A and B are mutually exclusive if

The definition extends naturally to sets. Two sets A and B are mutually exclusive ifor There is no intersection.

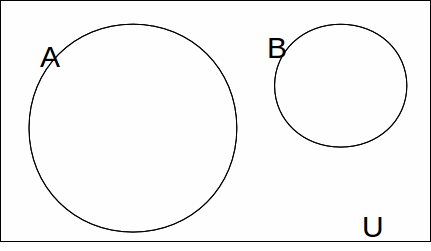
Events A and B are mutually exhaustive if either A must occur or B must occur or both. This then means thatIf events A and B are mutually exhaustive thenThis definition also extends naturally to sets. If sets A and B are mutually exhaustive then orIf A and B are also mutually exclusinve then

Events A and B are independent if event A does not affect the probability of event B and vice versa.andsatisfy the equationAlternatively, we can consider that event B has happened, and then consider the probability that A will happen. This is called 'conditional probability' – the probability of A happening conditional on B having happened, and is writtenThe equation for conditional probability is

If A and B are independent thenso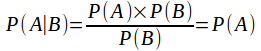which states the obvious fact that A is independent of B.