Introduction to Fourier Series

Basically, Fourier series express a function as a sum of sine or cosine terms. They are useful because many differential equations are linear, so that ifandare solutions to the equation then so isfor suitableandand by extension, any linear combination of solutions is also a solutions, with suitable coefficientExpressing a function as a sum of sine and cosine terms is especially useful because many wavefunctions are naturally expressed in terms of linear combinations of sine and cosine terms.

Any continuous function defined on an interval can be expressed as a Fourier series, and any function with a finite number of discontinuities. The function is extended outside the range on which it is defined by defining it as a repeating function, because sin and cosine are repeating functions with periodso for example, if

Then we can extendoutside the intervalby writingwhere

This is illustrated below.

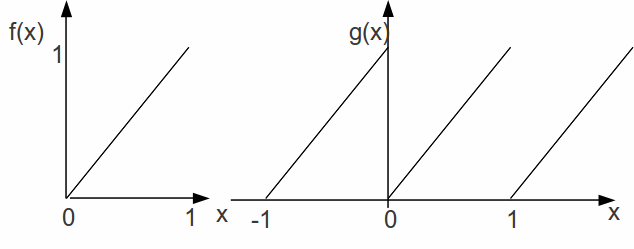
In general forWe can extendoutside the interval by writing

We can in fact extend a function in more than one way, besides the sample given above. We can extendto be an even function, so that

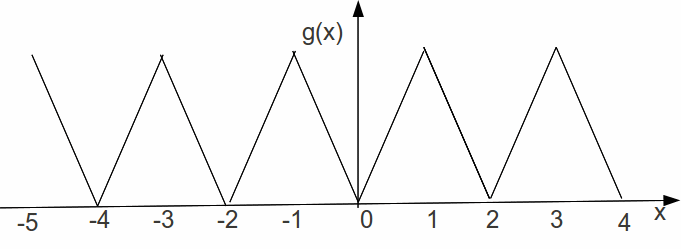
or we can extendto be an odd function, so thatwhile maintaining periodicity.

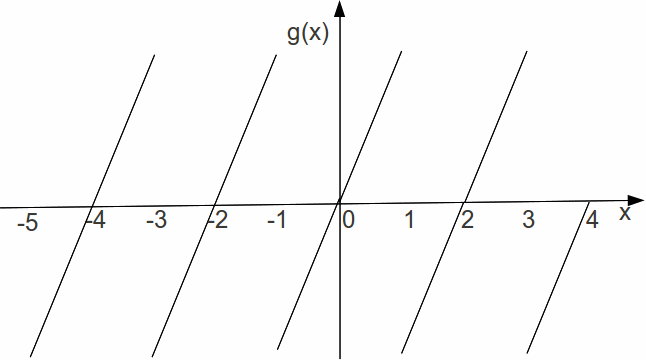
For these two extensions the period has doubled, but periodicity remains.