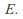The Weierstrass M - Test

Weierstrass's M – test provides a way to prove uniform convergence for the sum of a sequence.

Letbe a sequence of functions on a setand suppose that there is a sequence of positive termssuch that

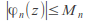1. for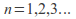and all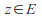

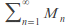2. is convergent

   Then the series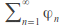is uniformly convergent on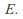

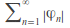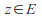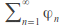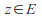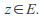   Proof: Note that by assumption 1 and 2,is convergent for eachby the comparison test. henceis convergent for eachby the absolute convergence test and so the sum functionexists for eachWe note the partial sum functions by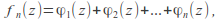

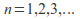
   Then for

   

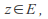
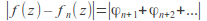
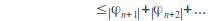   by the triangle inequality

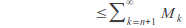   by assumption 1

   By assumption 2,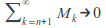as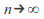so uniform convergence follows..

   Example: Prove that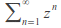converges uniformly on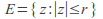for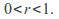

   

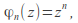
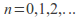
   and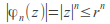for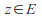

   so that assumption 1 above is satisfied with

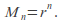
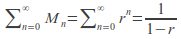   for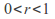

   so that assumption 2 is satisfied and the sum converges uniformly on