A Condition for Cauchy Sequences to Converge to the Same Limit

Theorem

Ifare Cauchy sequences in a metric spacesuch thatforthen

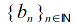1. is also a Cauchy sequence

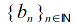2. converges to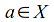if and only if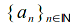converges to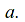

Proof

For 1:

Applying the triangle inequality,

LetWe can findsuch that

Sinceis Cauchy, there existssuch that

Now letwe gethence is Cauchy.

For 2:

Using the triangle theorem again gives

Hence,but

Ifthen and

Similarly, ifthen