Theorem
If
is a Haudorff space and if
is a finite subset of
thenis closed.
Proof
Let
and
A neighbourhood
of
exists such that
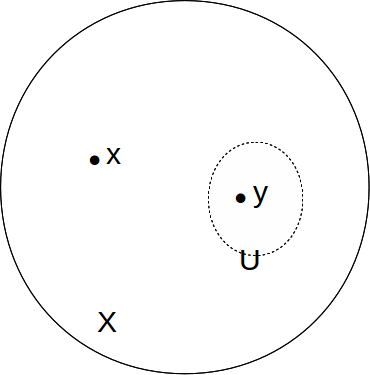
Hence
and
is open and
is closed.
By induction, any finite subset
is closed.
Call Us 07766496223
Theorem
Ifis a Haudorff space and ifis a finite subset ofthenis closed.
Proof
Letand
A neighbourhoodofexists such that
Henceandis open andis closed.
By induction, any finite subsetis closed.