Elastic strings are similar to springs. Both strings and springs obey Hooke's Law when being extended, but when being compressed, there is no tension in the string while the spring experiences a force tending to increase the length. (Springs obey Hooke's Law whether extended or compressed, but strings only obey Hooke's Law when extended).
The equation describing the tension is an extended string is
where
is the modulus of elasticity of the string
is the natural length of the string.
is the extension of the
Hooke's Law
is obeyed when the string is extended because we may write
We can find the energy stored in a stretched spring by integration.
Because
we may also write
Tension is a string is always directed towards the centre of the string, and has the same magnitude at each point of the string. If the string is fixed at one end to a surface with the other end free to move then we might consider only the end free to move. Typically this is attached to a mass or some other force. If a mass is hanging from the spring, then the spring will extend until the tension is equal to the force exerted by the mass (it's weight)
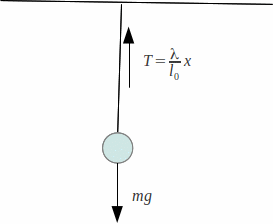
We may equate the forces to obtain