The fundamental equation connecting capacitance, voltage and charge is
Charge=Voltage*Capacitance (1)
If a battery is connected to a capacitor, the voltage across the capacitor will not instantly become the battery voltage. Instead current will flow in the circuit. Negative charge will collect on one plate and positive charge on the other. The voltage across the capacitor will build up and as it does the voltage available to drive the current through the internal resistance of the battery will decrease. Eventually after an infinite time, the voltage across the capacitor will reach the battery voltage and no current will flow. At each point during the charging of the capacitor equation (1) is obeyed. We can also related the voltage across the capacitor to the time elapsed since the battery was connected:
where
is the emf of the battery.
But then (1) gives the charge across the capacitor at any time
since if we multiply both sides by
we obtain
where
is the charge stored on the fully charged capacitor.
We can also find the current in the circuit at any time
by differentiating
obtaining
(2)
Now
Substituting this into (2) gives
But
is the initial current
so 
The qualitative graphs of
and
are shown below.
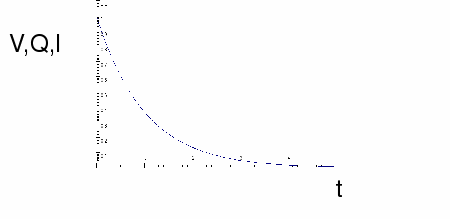
Similar arguments give the analogous equations for discharging capacitors.
and
The qualitative graph of V,Q and I against t is shown below, indicating exponential decay.
