Some probabilities are especially suitable to calculate from tables. If we have two events with a range of possible outcomes then we can display these in a table and use the table to calculate probabilities. Suppose for example that we have two six sided fair dice. Each dice is equally likely to score anything from one to six, and we find the total score by adding the scores on the dice. We can draw up the table below. The sum of the scores on the dice is highlighted in green.
|
+ |
Dice 2 |
||||||
|
1 |
2 |
3 |
4 |
5 |
6 |
||
|
Dice 1 |
1 |
2 |
3 |
4 |
5 |
6 |
7 |
|
2 |
3 |
4 |
5 |
6 |
7 |
8 |
|
|
3 |
4 |
5 |
6 |
7 |
8 |
9 |
|
|
4 |
5 |
6 |
7 |
8 |
9 |
10 |
|
|
5 |
6 |
7 |
8 |
9 |
10 |
11 |
|
|
6 |
7 |
8 |
9 |
10 |
11 |
12 |
|
Presented in this way we can easily find
-
The probability of scoring 3 or less. There is a 2 and two 3's so there are three ways of scoring 3 or less and 6*6=36 possible combinations or scores.. The probability is
-
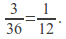
The probability of scoring a 7 is
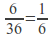since there are six 7's in the table. -
The probability of not scoring a five. There are five 5's in the table so the probability of scoring a five is 5 over 36 and the probability of not scoring a five is
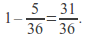Alternatively we could count the number of scores other that five in the table. There are 31 of them so the probability is
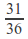as before.