
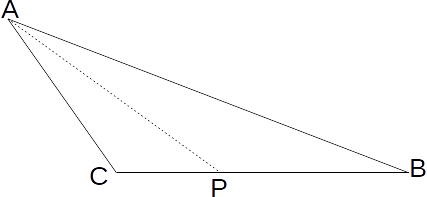
\[\frac{1}{2} PC \times PA \times sin (\angle APC)\]
.The are of APB is
\[\frac{1}{2} PB \times PA \times sin (\angle AP)\]
.The ratio of the areas is
\[\frac{1}{2} PC \times PA \times sin (\angle APC): \frac{1}{2} PB \times PA \times sin (\angle AP)\]
.\[PC \times sin (APC):PB \times sin (\angle AP)\]
.But
\[ sin (\angle APC)=sin (\angle AP)\]
since \[\angle APC+ \angle APB=180\]
.Hence the ratio of the areas of the triangles is
\[PC :PB\]
. 