\[h\]
and base radius \[r\]
is \[V=\pi r^2 h\]
.A frustum is a truncated cone. Part of the top is cut off by a cut parallel to the base.
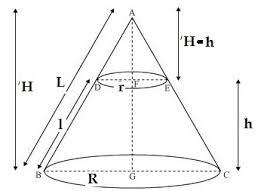
\[\frac{H-h}{r}=\frac{H}{R} \rightarrow HR-hR=Hr \rightarrow H=\frac{hR}{R-r}\]
and \[H-h=\frac{hR}{R-r}-h=\frac{hR-hR+hr}{R-r}=\frac{hr}{R-r}\]
.The volume of the frustum is then
\[\begin{equation} \begin{aligned} V_{FRUSTUM}&=\frac{1}{3}\pi R^2H-\frac{1}{3}\pi r^2(H-h) \\ &=\frac{1}{3}\pi(R^2H-r^2(H-h)) \\ &=\frac{1}{3}\pi(R^2(\frac{hR}{R-r})-r^2 (\frac{hr}{R-r})) \\ &=\frac{1}{3(R-r)}\pi (R^3-r^3) \end{aligned} \end{equation}\]
