A regular Sturm Liouville equation is a real second-order linear differential equation of the form
with boundary conditions
with at least one of
and similarly for
and with the functions
specified.
In the simplest case all coefficients are continuous on the finite closed interval
and
has continuous derivative. In this case, this function
is a solution if it is continuously differentiable on
and satisfies (1) at every point in
In addition,is typically required to satisfy some boundary conditions at
and
The function
is called the weight function.
The value of
is not specified in the equation; finding the values offor which there exists a non-trivial solution of (1) satisfying the boundary conditions is part of the problem called the Sturm–Liouville problem.
Such values ofwhen they exist are called the eigenvalues of the boundary value problem defined above and the given boundary conditions. The corresponding solutions (for each) are the eigenfunctions of this problem. The solutions (eigenfunctions) of each Sturm - Liouville problem form an orthogonal basis for the set of continuous functions, so that if
and
are solutions for distinct
then
We wish to find a function
which solves the following Sturm – Liouville problem:
with
In fact
is a solution with eigenvalue
Properties:
The eigenvalues of Sturm – Liouville problems form an infinite set:
with 
as
Any pair of eigenfunctions corresponding to a particular eigenvalue are non – zero multiples of one another.
If
is a complete set of eigenfunctions for (1) then
a) The
form a basis for
the set of continuous functions with continuous first derivatives.
b)Let
be any function in
and let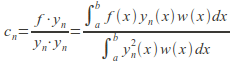
be the nth Fourier coefficient ofwith respect to the basis
then the Fourier series
converges pointwise toon