The Harmonic series is the infinite series whose sum is given by
The harmonic series diverges. This is quite easy to prove.
Thus
is unbounded so
is divergent.
In fact
is in many cases divergent for many
for example if
S is the set of prime numbers
S is the set of multiples of any number.
The series diverges very slowly. For example, the sum of the first
terms is less than 100. This is because the partial sums of the series have logarithmic growth:
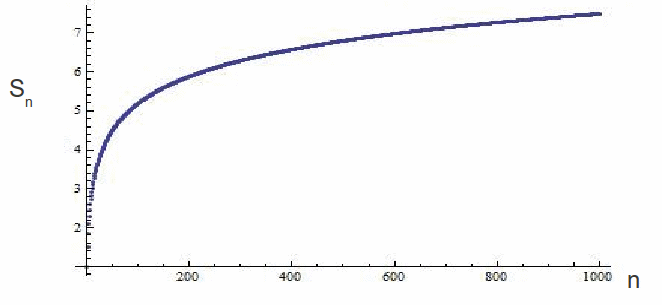
as can easy be proved using the integral test.