Differentiating Under the Integral Sign

Let R be a region and let K be a complex valued function of two variables z in R and t in [a,b] such that

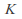1. is analytic in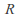as a function of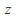for each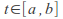

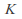2. and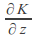are continuous on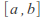as functions of t for each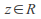

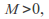
3. For some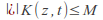for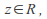

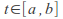
Then the functionwithis analytic onandfor(1)

Proof: Letand choose a circleinwith centreand radiussuch that the inside oflies entirely inIflies insidethen we have by assumption 1 and Cauchy's Integral Formula,

and

and by Cauchy's First Derivative Formula,for each t in [a,b].

Hence, if f is given by (1) then

say. We need to show thatas

henceas