Suppose that a complex valued function
operating on complex numbers has a singularity at
Then
-
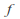has a removable singularity at
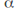if there is a function
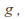analytic at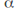such that
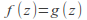for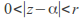
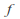- has a pole of order
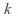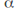atif there is a function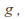analytic at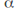with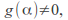 
and a positive number
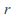such that
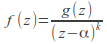for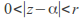 - 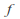has an essential singularity at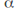if the singularity at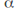is neither removable or a pole.
Example:
has a singularity at 0 since the denominator =0 at
but the singularity is removable since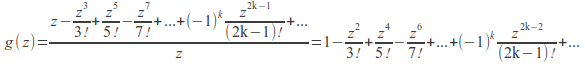
is analytic atand
on
Example:
has two poles, one atand one at
so the pole at
has order 1.
so the pole athas order 2
Example:
has an essential singularity at
To see this, write
Because the powers of
are negative and becoming larger without limit, the singularity is essential. We cannot multiplyby
for anyto make
analytic.